I will give you $1,000.
[editor’s note – This is hypothetical, please do not email me to collect any money]
Do you want the $1,000 today or the $1,000 in 1 year?
You want that money today, right? Time value of money is the concept that you would rather have money today than the same amount in the future.
End of Post, concept explained, thanks for reading……..
You are still here… I guess that means you want a bit more. Allright. You could invest that money and earn interest, so even if you put that $1,000 in a savings account earning a whopping 0.01%, it would still earn 10 cents of interest by year end. Check my math but $1,000.10 is greater than $1,000 and you would be better off taking the money today.
Or, it you use the money to pay down student loan balance at 3.5% interest instead, you would save $35 in interest over just 1 year. The better the opportunities you have, the more you would want the money today.
Time Value of Money is the concept that cash today is worth more than cash in future
Now what if I told you I will give you $1,000 today or $1,100 at the end of the year? $1,100 is higher than $1,000. You only need to wait 1 year. Which option do you choose now?
If I have $1,000 and can earn a risk-free 10% return over the next year, what does $1,000 end up being at the end of the year?
HA – trick question, the answer is zero, my wife spent it at Target. But what if you had the same opportunity…
Example 1: Future Value Calculation for 1 Time Period
Your $1,000 invested at a 10% return would grow to $1,100. The formula shows how to calculate the value in one year of $1,000 invested at 10%. You simply multiply $1,000 by (1 + 10%). Therefore, you would be indifferent to the offer of getting $1,000 today or $1,100 in a year.
The great thing about the concept is that it works in reverse as well. If you know you are getting $1,100 in a year, you can find out what the equivalent money needed today would be given you could earn 10% on it.
Example 2: Present Value Calculation for 1 Time Period
As you can see, $1,100 a year away is worth $1,000 in the present day when using a 10% growth rate. If you look at the formula you can see that when you grow an investment you take the value multiplied by (1+ rate). If you want to discount a future value back you divide it by (1 + rate).
Should be fairly straightforward to this point.
Now what if instead of 1 year, you are looking over a 2 year period? Clearly, you would want something more than $1,100 in year 2 since it is even further away.
Example 3: Time Value of Money over 2 Time Periods
We know that $1,000 grown at 10% gives us $1,100 in 1 yr. $1,100 x (1+10%) will result in $1,210 at the end of the second year. The reverse is true again. The present value of $1,210 given to you 2 years in the future would result in $1,000 today assuming a 10% annual rate.
Wouldn’t it be easier if you could do it in 1 step though? If you had to do 20 separate calculations to discount 20 years, imagine how cumbersome it would be. That would not be efficient. Luckily, (1+10%) x (1+10%) = (1+10%)^2 as shown below. Notice, nothing changed except being able to discount 2 years in only 1 step.
Example 4: Time Value of Money over 2 Time Periods – in one step
All the above is simple, but what we now know is:
You can calculate a Future Value any number of time periods in the future by multiplying it by (1+return%)^n
You can calculate a Present Value any number of time periods in the past by dividing it by (1+return%)^n
You can find the value at any point in time of any cashflow stream
What if you were offered $500 in 1 year and $100 in 2 years or you could choose $800 in 4 years? You have the tools:
Example 5: Time Value of Money over Multiple Payments
$500 invested for 3 years and $100 invested for 2 years will result in $787 total.
Therefore you would be better off taking $800 in year 4.
What is the value today of $500 in 1 year and $100 in 2 years?
You could take $500 and $100 cashflows divided by 1.1 and 1.1^2 respectively OR you can take the Future value of $787 and divide it by 1.1^4. Either way gets the same answer of $537 today. As long as the cashflows and the rate stays the same, you can find the value at any point in time by using the future and present value calculations above.
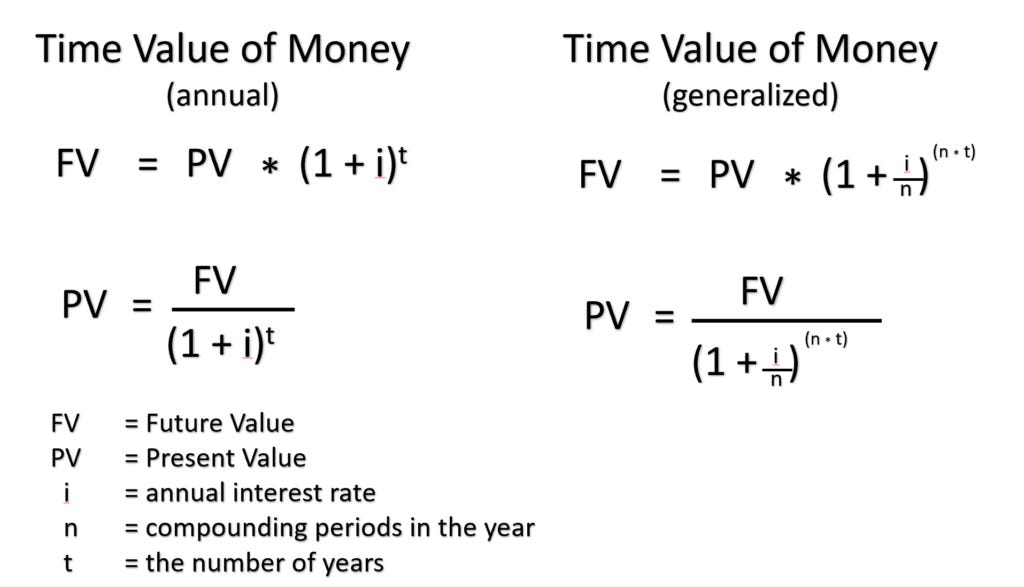
Where are the Formulas?
We have been working in annual timesteps, but you can receive a cashflow at any time. The generalized formulas cover non-annual cases
You wanted formulas, now you got them. Now lets dig into compounding briefly. When you make an investment, you can get paid the earnings at different periods (ie- annual, monthly, daily, etc). The thing to remember is the more frequent the compounding is, the higher the ending value will be. Why? You get to earn a return on your previous earnings. For example, annual compounding, you get all your return at the end of the year. You invest $1,000 and for 364 days you have $1,000. Then you get your $100 of return and have $1,100.
But, what if you received your earnings semi-annual? At the end of 6 months, you would get $50 of earnings (10% rate divided by half is 5% per half-year). What do you do with that $50? You invest it. Now you have $1,050 invested and the $1,050 will return $52.50 over the next 6 months. You end the year at $1,102.50 due to that extra compounding.
In the general, the more frequent the compounding the higher the return
This is over a one year period. Over longer time periods the differences are even more pronounced.
Why is Time Value of Money Important?
This is a foundational concept across all of finance and business. Time value of money calculations are nearly always involved when a business owner has to value a project or decide on a project to choose. Similarly, the present value of cashflows is the entire concept behind the “discounted cash flow” (DCF) method used in valuing a stock. This underpins concepts like payback period, internal rate of return, and value of business.
Time value of money is also a concept poorly understood and underutilized in the personal finance space. The decision on college should be based largely around tradeoffs using time value of money, similar to how a business decides if it is worth the cost to invest in a project.
If you are investing in real estate and have 2 houses you have to choose between that are different costs and produce different rental streams, which do you buy? Now you have the foundational knowledge to figure it out.
Anything Else You Should Know?
I used a 10% return throughout this post. However, choosing what rate to use in your time value of money calculation is a entire post to itself. In short, you want to choose a return that matches up with your opportunity cost. Opportunity cost is the ‘cost’ of the next best choice. In the rental example, you could use the mortgage rate as your return (more likely mortgage rate + some additional profit).
Additionally, the more risky an investment, the higher the rate should be. Imagine 2 investments with the same exact cost and future cashflows, but investment A is with a shady company that is likely to scam you and investment B is with a trustworthy company If you use the same rate, the present value of the projects is the same and you would be indifferent. However, you should demand a higher profit from the riskier investment. The higher the rate used, the lower the present value of a cashflow is. Now Investment B is the better choice













Love that you’re growing a small army of actuaries in training w the stack here.
Quick heads up that substack ate your exponents in the top half of the post so that 1.1^4 gets shown as just 1.14